PDF(2340 KB)
Research on the Crescent-rib Bifurcation with Waistline Turning Angle of Zero Degree
Bi-fei WANG, Shao-jun XIONG, Qi-hang WANG, Yue-wei LI
PDF(2340 KB)
Research on the Crescent-rib Bifurcation with Waistline Turning Angle of Zero Degree
The three-dimensional finite element method is used to analyze the stress of crescent-rib bifurcation with waistline turning angle of zero degree and the influence of waistline turning angle on the stress of the wall and crescent-rib of bifurcation. The results indicate that waistline turning angle has little effect on the stress of the crescent-rib. The design scheme with waistline turning angle of zero degree reduces the stress in the obtuse angle area and improves the stress of the bifurcation. Keeping the wall thickness of basic cone unchanged， the ability of bifurcation to bear internal pressure can be improved by increasing the wall thickness of transition pipe. Because the waistlines of the main cone pipe and the branch cone pipe are collinear， the fabricating difficulty and error can be appropriately reduced during assembly， which can be used in engineering design.
wall of bifurcation / crescent-rib / finite element method / waistline turning angle / stress of Z direction {{custom_keyword}} /
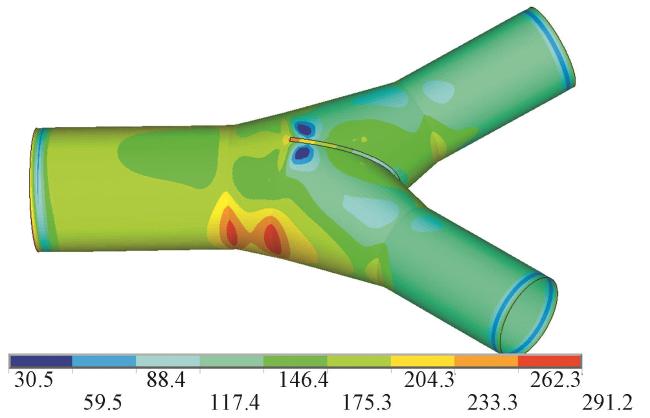
Fig.4 Mises stress of bifurcation with waistline turning angle of 9.9°图4 腰线折角为9.9°的管壁Mises应力云图（单位：MPa） |
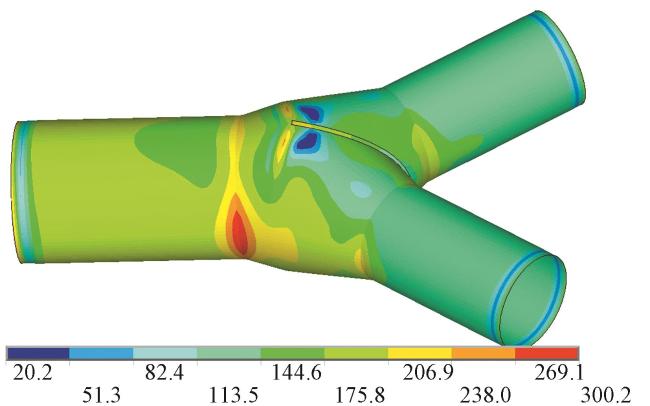
Fig.5 Mises stress of bifurcation with waistline turning angle of 0°图5 腰线折角为0°的管壁Mises应力云图（单位：MPa） |
Fig.7 Relationship curve of Mises stress of Point A and waistline turning angle图7 岔管管壁关键点A的Mises应力与腰线折角关系曲线 |
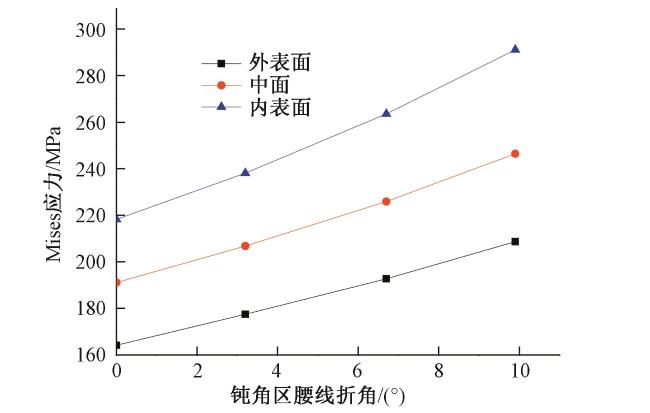
Fig.8 Relationship curve of Mises stress of Point B and waistline turning angle图8 岔管管壁关键点B的Mises应力与腰线折角关系曲线 |
Fig.9 Relationship curve of Mises stress of Point C and waistline turning angle图9 岔管管壁关键点C的Mises应力与腰线折角关系曲线 |
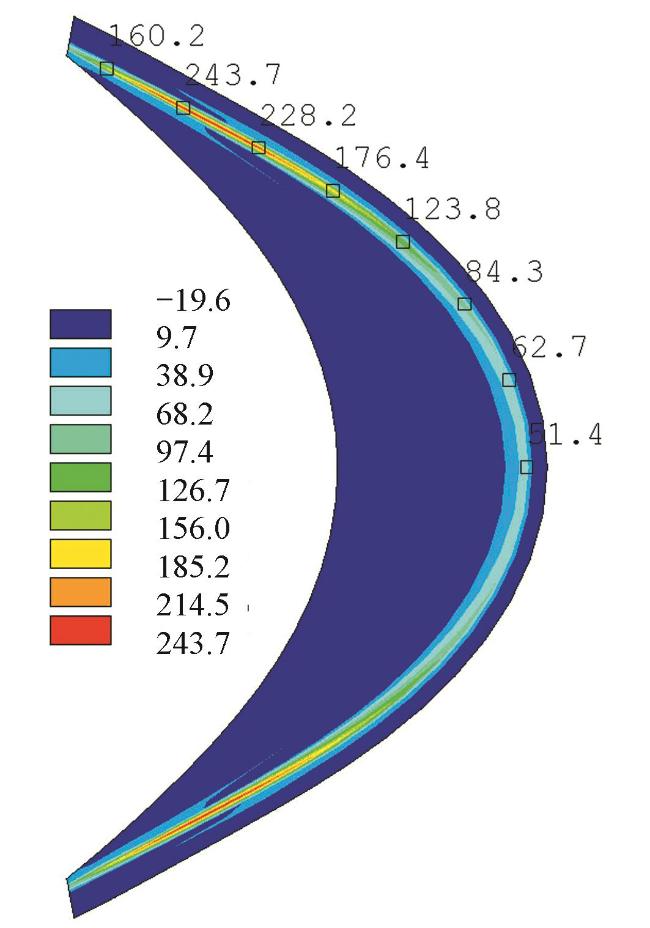
Fig.11 Stress of Z direction of crescent-rib with waistline turning angle of 0°图11 腰线折角为0°的月牙肋Z向应力云图（单位：MPa） |
Fig.12 Mises stress of crescent-rib with waistline turning angle of 0°图12 腰线折角为0°的月牙肋Mises应力云图（单位：MPa） |
| 1 |
马善定，伍鹤皋，秦继章. 水电站压力管道［M］. 武汉： 湖北科学技术出版社， 2002.
{{custom_citation.content}}
{{custom_citation.annotation}}
|
| 2 |
伍鹤皋，石长征，苏凯.埋藏式月牙肋钢管结构特性研究［J］. 水利学报， 2008，39（4）：460-465.
{{custom_citation.content}}
{{custom_citation.annotation}}
|
| 3 |
汪碧飞，李勇泉，陈美娟， 等. 埋藏式月牙肋岔管内压分担比研究［J］. 长江科学院院报， 2021，38（6）：123-127.
{{custom_citation.content}}
{{custom_citation.annotation}}
|
| 4 |
申燕，李晓，邵艳妮，等. 月牙肋钢岔管结构优化及联合承载机理研究［J］. 中国农村水利水电， 2016（4）：154-157.
{{custom_citation.content}}
{{custom_citation.annotation}}
|
| 5 |
汪碧飞，颜家军，孔剑， 等. CATIA在月牙肋钢岔管三维参数化设计中的应用［J］. 水利水电快报， 2018，39（12）：19-21.
{{custom_citation.content}}
{{custom_citation.annotation}}
|
| 6 |
田静，楚万强，许性永，等. 埋藏式月牙肋钢钢管结构三维有限元分析［J］. 水电能源科学， 2011，29（5）：86-88.
{{custom_citation.content}}
{{custom_citation.annotation}}
|
| 7 |
中华人民共和国水利行业标准. SL/T 281 -2020， 水利水电工程压力钢管设计规范 ［S］.北京：中国水利水电出版社， 2020.
{{custom_citation.content}}
{{custom_citation.annotation}}
|
| 8 |
乔淑娟，罗京龙，伍鹤皋. 月牙肋岔管体形优化与设计［J］. 中国农村水利水电， 2004（12）：116-118.
{{custom_citation.content}}
{{custom_citation.annotation}}
|
| 9 |
杨海霞，李哲斐. 月牙肋岔管的优化与设计［J］. 固体力学学报， 2006，27（12）：180-183.
{{custom_citation.content}}
{{custom_citation.annotation}}
|
| 10 |
申燕，杨鑫，蒋逵超.月牙肋岔管肋板内缘曲线对结构受力特性的影响［J］. 西北水电， 2002（3）：56-62.
{{custom_citation.content}}
{{custom_citation.annotation}}
|
| 11 |
杜芳琴，伍鹤皋，石长征. 月牙肋钢岔管设计中若干问题的探讨［J］. 电能源科学， 2012，30（8）：129-131.
{{custom_citation.content}}
{{custom_citation.annotation}}
|
| 12 |
汪碧飞. 一种腰线为零的对称钢岔管［P］. 中国专利： 202022367485.1， 2021-08-06.
{{custom_citation.content}}
{{custom_citation.annotation}}
|
| 13 |
苏凯，李聪安，胡馨之，等. 埋藏式月牙肋钢岔管肋板受力特性和体型优化方法［J］. 天津大学学报（自然科学与工程技术版）， 2018，51（3）：232-240.
{{custom_citation.content}}
{{custom_citation.annotation}}
|
| 14 |
王志国. 关于内加强月牙肋岔管肋板用钢材Z向性能基本选择的初步探讨［C］// 第7届全国水电站压力管道学术会议论文集. 北京：中国水利水电出版社， 2010：268-272.
{{custom_citation.content}}
{{custom_citation.annotation}}
|
| 15 |
中华人民共和国国家标准. GB/T 5313 -2010， 厚度方向性能钢板 ［S］.北京：中国标准出版社，2011.
{{custom_citation.content}}
{{custom_citation.annotation}}
|
| {{custom_ref.label}} |
{{custom_citation.content}}
{{custom_citation.annotation}}
|
PDF(2340 KB)
Fig.1 Crescent-rib bifurcationFig.2 Size and key points of bifurcation with waistline turning angle of 0°Fig.3 Finite element model of bifurcation with waistline turning angle of 0°Fig.4 Mises stress of bifurcation with waistline turning angle of 9.9°Fig.5 Mises stress of bifurcation with waistline turning angle of 0°Fig.6 Mises stress of key points of wall of bifurcationFig.7 Relationship curve of Mises stress of Point A and waistline turning angleFig.8 Relationship curve of Mises stress of Point B and waistline turning angleFig.9 Relationship curve of Mises stress of Point C and waistline turning angleFig.10 Relationship curve of Mises stress of Point D and waistline turning angleFig.11 Stress of Z direction of crescent-rib with waistline turning angle of 0°Fig.12 Mises stress of crescent-rib with waistline turning angle of 0°Fig.13 Relationship curve of external Mises stress of crescent-rib and waistline turning angle
鄂公网安备 42010602001274号 鄂ICP备14015464号-1/
| 〈 |
|
〉 |
