云南降水的5个统计指标及其空间分布

刘有梅, 王龙, 余航, 和梅辉, 高瑞, 王葵

PDF(4057 KB)
节水灌溉
中国农村水利水电 ›› 2021 ›› (3) : 80-87.
水文水资源

云南降水的5个统计指标及其空间分布

作者信息 +

Five Statistical Indicators of Precipitation in Yunnan and Their Spatial Distribution

Author information +
稿件信息 +

摘要

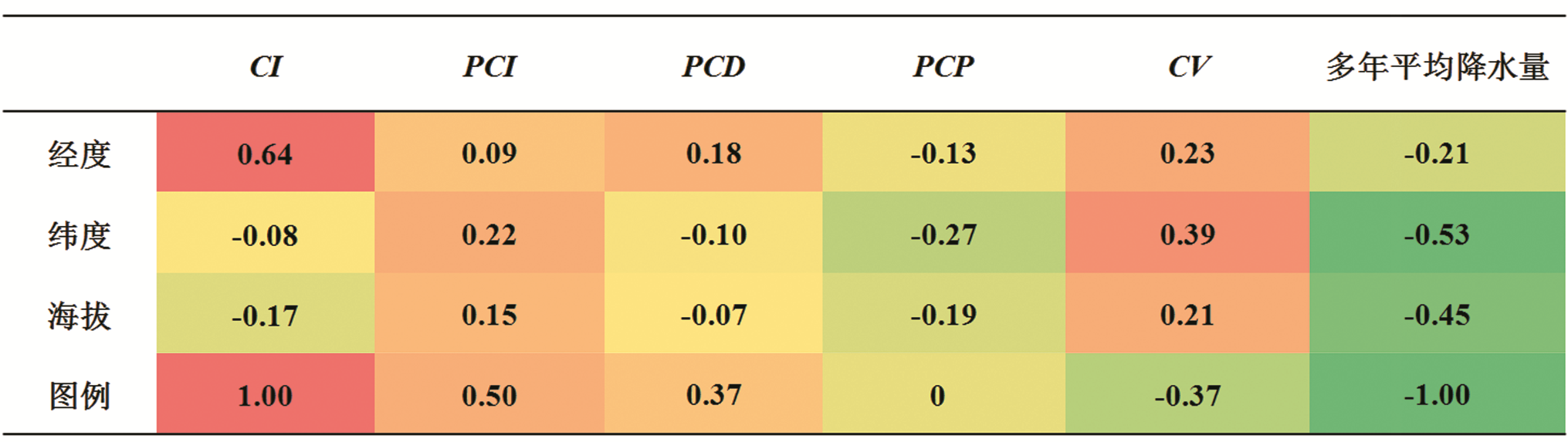
利用云南29个气象站1960-2014年的日降水数据，计算集中指数（Concentration Index，CI）、降水集中指数（Precipitation Concentration Index，PCI）、降水集中度（Precipitation Concentration Degree，PCD）、降水集中期（Precipitation Concentration Period，PCP）、变差系数（Coefficient of Variation，CV）5个统计指标，分析了各指标之间的关系，并讨论了各指标的空间分布。结果表明：①CI介于0.62~0.71之间，平均为0.67，日降水高度集中。CI与年降水量、降水日数的相关系数分别为-0.50、-0.93，具有显著的负相关关系（α=0.05），年降水量和降水日数较少的地区，CI较大。CI与经度的相关系数为0.64，具有显著的正相关关系（α=0.05）。②PCI介于11.95~21.48之间，其中，3%的站点降水高度集中，52%的站点降水集中，45%的站点降水中度集中。PCD介于0.30~0.74之间，滇西北和滇东南地区的PCD较小。PCP的分析结果表明，云南降水主要集中在7、8月份。③CI和PCI、PCD分别关注降水的等级分布和集中程度，PCP则关注集中的时间段。PCI与PCD的空间分布情况基本上是一致的，且二者的相关系数为0.90，具有显著的正相关关系（α=0.05），故二者在一定程度上可以相互替换。采用CI、PCI和PCP 3种指标可以较好地描述降水的年内等级分布、集中程度和集中时段。④CV介于0.11~0.21之间，降水的年际变化随着纬度的减小、年降水量的增多呈减小的趋势，相关系数分别为0.39、-0.74；而年降水量随着海拔和纬度的升高呈下降的趋势，相关系数分别为-0.45、-0.53。

Abstract

Based on the daily precipitation data of 29 meteorological stations in Yunnan from 1960 to 2014， five statistical indexes are calculated， including the Concentration Index （CI）， Precipitation Concentration Index （PCI）， Precipitation Concentration Degree （PCD）， Precipitation Concentration Period （PCP） and Coefficient of Variation （CV）. The relationship between each index is analyzed， and the spatial distribution of each index is discussed. The results show that ① CI ranges from 0.62 to 0.71， with an average of 0.67， and the daily precipitation is highly concentrated. The correlation coefficients between CI and annual precipitation and precipitation days are -0.50 and -0.93， respectively， which have a significant negative correlation （α=0.05）. CI is larger in areas with less annual precipitation and precipitation days. The correlation coefficient between CI and longitude is 0.64， which has a significant positive correlation （α=0.05）. ② PCI ranges from 11.95 to 21.48， in which 3% of the stations are highly concentrated， 52% of the stations are concentrated， and 45% of the stations are moderately concentrated. PCD ranges from 0.30 and 0.74， and PCD in northwestern and southeastern Yunnan is smaller than that in other parts of Yunnan. The results of PCP analysis show that the precipitation in Yunnan is mainly concentrated in July and August. ③ CI， PCI and PCD pay attention to the grade distribution and concentration degree of precipitation respectively， while PCP pays attention to the period of concentration. The spatial distribution of PCI and PCD is basically the same， and the correlation coefficient between the two is 0.90， which has a significant positive correlation （α=0.05）， so they can replace each other to some extent. The annual grade distribution， concentration degree and concentration period of precipitation can be well described by using CI， PCI and PCP. ④ CV is between 0.11 and 0.21， the interannual variation of precipitation decreases with the decrease in latitude and the increase in annual precipitation， the correlation coefficient are 0.39 and -0.74 respectively， while the annual precipitation decreases with the increase of elevation and latitude， the correlation coefficient are -0.45 and -0.53 respectively.

关键词

统计特征 / 降水 / 空间分布 / 云南

Key words

statistical characteristic / precipitation / spatial distribution / Yunnan

基金

国家自然科学基金(51669035)
云南省教育厅基金(2020J0246)

引用本文

EndNote

Ris (Procite)

Bibtex

导出引用
刘有梅 , 王龙 , 余航 , 和梅辉 , 高瑞 , 王葵. 云南降水的5个统计指标及其空间分布[J].中国农村水利水电, 2021(3): 80-87
You-mei LIU , Long WANG , Hang YÜ , Mei-hui HE , Rui GAO , Kui WANG. Five Statistical Indicators of Precipitation in Yunnan and Their Spatial Distribution[J].China Rural Water and Hydropower, 2021(3): 80-87
降水变化对工程建设、生产生活、灾害预防等工作至关重要，近年来，研究者们将降水的变化特征分为时间和空间两方面来研究。时间上，年内降水集中程度过高和集中时间过长，容易导致干旱、滑坡及洪涝灾害的发生［1-3］、影响最佳的农业灌溉时间［4］，导致农业或其他财产损失和人员伤亡［5］。年际降水分布不均匀，将影响农业、水资源的管理及综合规划［6，7］。为此，研究者们提出了很多统计指标来描述降水的年内、年际特征并被广泛运用。其中，用以描述年内分布特征的指标主要分为两类，一类是基于日降水数据统计降水天数、不同等级降水量对总降水量的贡献，以Martin-Vide（2004）的集中指数（Concentration Index，CI）为代表；第二类是基于月降水数据统计降水年内分布的不均匀性，以Oliver（1980）、Michiels等人（1992）的降水集中指数（Precipitation Concentration Index，PCI）及张录军等人（2003）的降水集中度（Precipitation Concentration Degree，PCD）、降水集中期（Precipitation Concentration Period，PCP）为代表。空间上，常用上述指标的空间分布图来描述各地区降水的差异。针对以上特征，有的研究者利用CI指标来分析各地区日降水集中的时空分布［8-12］、分布成因［13］、气候变化［14］及其与大气环流的关系［15］；有的研究者利用PCI、PCD、PCP指标来分析各地区月降水的集中程度和集中时段［3，16-22］。而为了比较降水在年际间的差异性，大多数研究者常采用变差系数（Coefficient of Variation，CV）来描述降水的年际分布特征。如：Royé将变差系数CV结合CI指标共同解释了美国相邻地区CI的空间分布模式［23］。
综上所述，之前的研究者分别用各降水统计指标分析了世界各地的降水年内、年际统计特征及空间分布，如印度［14］、美国［23］、俄罗斯［11］、智利［12］、中国［13，15，24］等。然而，很少有研究者将上述所有指标结合起来共同分析降水的年内、年际统计特征及空间分布，并讨论它们的关系。因此，本文利用云南29站数据，首先在计算上述指标的基础上，分析了各指标之间的关系，并讨论了各指标的空间分布特征。

1 数据和方法

1.1 研究区域及数据来源

云南地处中国西南，平均海拔在600~3 000 m之间，经纬度在97°31′~106°11′E，21°8′~29°15′N之间。本文选取了降水系列较长、观测记录较为完整的29个气象站1960-2014年的日降水数据来分析该地区降水的年内、年际统计特征。其中，选取的气象站海拔在400.9~3 319 m之间，经、纬度分别在97°51′~105°04′E，21°29′~28°29′N之间（如图1所示）。数据来源于中国气象局国家气象科学数据共享服务平台（http：//data.cma.cn）。
图1 气象站点示意图

Fig.1 Locations of meteorological stations

Full size|PPT slide

1.2 方 法

本次研究用于分析降水的年内、年际统计特征及空间分布的指标包括CI、PCI、PCD、PCP、CV，其计算方法如表1所示。
表1 降水统计指标的计算方法

Tab.1 Calculation method of precipitation statistical index

指标 CI PCI PCD、PCP CV
定义 基于日降水数据定义一定时期内降水发生在某个降水等级的频数，分析降水在某个地区各个降水等级的年内分布特征及降水的极端程度。 基于月降水数据定量地描述降水模式在时间及季节上的相对分布，量化降水在某地区的年内分布。 引入向量特征，基于月降水数据来分析降水年内的时间分配特征，量化降水的年内集中程度。 反映年降水量的年际变化规律，能够表征某一地区降水的年际变化程度。CV的大小反映了年降水量的逐年变化的相对大小。

计算

公式

CI= 1-[abebxx-1b]01005 000

ln a=∑Xi2∑lnYi+∑Xi∑XilnXi-∑Xi2∑lnXi-∑Xi∑XilnYiN∑Xi2-(∑Xi)2

b=N∑XilnYi+∑Xi∑lnXi-N∑XilnXi-∑Xi∑lnYiN∑Xi2-(∑Xi)2

其中，a、b采用最小二乘法计算，N代表类数。

PCI=100× ∑i=112Pi2(∑i=112Pi)2

Pi为第i月的降水量。

PCD= Rxi2+Ryi2/Ri

PCP= arctan Rxi/Ryi

Rxi=∑rij∙sinθj ；

Ryi=∑rij∙cosθj ;

式中：Ri 表示某测站第i年的总降水量；rij 为第i年第j月的总降水量； θj为第j月对应的方位角。

CV= σR = 1R1n-1∑Ri-R2

式中：n为资料年数；Ri 为各年的年降水量；R为多年降水总量的平均值。

特点 将降水分多个等级，评价了不同等级的降水量对总降水量的贡献。 计算简便、物理意义直观。可划分为四个等级来表征年内降水的集中程度，如表3所示，但不能明确表征降水的集中时段。 表征年内降水的集中程度，明确年内降水集中的具体时段。其中，PCD越接近1，年内降水分布越集中。 通过标准差和平均值的比率来消除变量的维数，即在计算时消除了测站本身降水的差异性，常和其余几个降水统计指标一起使用来共同表征降水的年内、年际特征。

2 结果与分析

根据表1的计算方法，计算得到云南29站CI、PCI、PCD、PCP、CV 5个指标，结果见表2。
表2 云南省各站点的地理信息及各指标计算结果（1960-2014年）

Tab.2 Geographic information of each site in Yunnan Province and calculation results of various indicators (1960-2014)

台站 CI PCI CV

多年平均降水量/

mm

 经度/(°) 纬度/(°) 海拔/m

集中期/

月

 Ni /% PCD
广南 0.70 15.45 0.15 1 016.44 105.07 24.07 1 249.60 7.11 20.42 0.53
屏边 0.70 14.62 0.13 1 624.05 103.68 22.98 1 414.10 7.73 22.69 0.53
蒙自 0.68 15.54 0.18 840.55 103.38 23.38 1 300.70 7.72 24.99 0.52
江城 0.62 15.64 0.11 2 233.93 101.85 22.58 1 120.50 8.07 28.79 0.57
勐腊 0.67 15.49 0.15 1 517.11 101.57 21.48 631.90 7.76 26.59 0.55
元江 0.71 15.35 0.20 794.85 101.98 23.60 400.90 7.71 24.25 0.49
思茅 0.65 16.52 0.14 1 487.37 100.97 22.78 1 302.10 8.05 27.53 0.59
景洪 0.69 15.39 0.15 1 156.45 100.78 22.00 582.00 7.99 24.40 0.54
澜沧 0.63 16.20 0.12 1 590.56 99.93 22.57 1 054.80 8.16 29.16 0.60
临沧 0.63 16.20 0.14 1 145.58 100.08 23.88 1 502.40 8.26 29.22 0.59
泸西 0.68 15.86 0.18 906.17 103.77 24.53 1 704.30 7.71 25.43 0.54
玉溪 0.67 16.06 0.18 890.80 102.55 24.35 1 636.80 7.81 25.77 0.55
景东 0.68 16.08 0.16 1 108.01 100.87 24.47 1 162.30 8.00 25.11 0.57
瑞丽 0.64 16.59 0.15 1 406.62 97.85 24.02 776.60 7.92 27.91 0.61
沾益 0.69 16.17 0.19 968.70 103.83 25.58 1 898.70 7.73 24.89 0.54
昆明 0.68 17.05 0.20 984.50 102.68 25.02 1 893.40 7.94 25.43 0.60
楚雄 0.68 17.95 0.21 850.02 101.55 25.03 1 824.10 7.86 24.99 0.62
元谋 0.69 18.82 0.19 632.07 101.87 25.73 1 120.60 8.03 24.71 0.65
大理 0.70 16.48 0.19 1 045.28 100.18 25.70 1 990.50 8.21 23.73 0.56
保山 0.67 15.37 0.16 970.37 99.17 25.12 1 653.50 8.00 25.65 0.51
腾冲 0.62 15.19 0.14 1 478.00 98.50 25.02 1 654.60 8.01 29.37 0.55
会泽 0.68 17.12 0.17 781.88 103.28 26.42 2 110.50 7.85 25.50 0.61
华坪 0.68 21.48 0.19 1049.88 101.27 26.63 1 230.80 8.20 25.76 0.74
丽江 0.63 20.00 0.15 960.08 100.22 26.85 2 380.90 8.30 28.33 0.71
昭通 0.69 16.79 0.17 693.27 103.72 27.35 1 949.50 7.75 25.74 0.60
维西 0.65 14.53 0.15 946.72 99.28 27.02 2 326.10 7.08 26.90 0.36
中甸 0.64 18.47 0.16 629.60 99.70 27.83 3 276.70 8.16 28.98 0.60
贡山 0.63 11.95 0.15 1 707.74 98.68 27.75 1 583.30 7.21 28.72 0.30
德钦 0.67 15.17 0.20 637.24 98.92 28.48 3 319.00 7.03 26.46 0.47

2.1 基于日降水的年内统计特征

根据表2，29站CI介于0.62~0.71之间，元江站CI最高（CI=0.71），腾冲站CI最低（CI=0.62），平均为0.67。根据Martin-Vide（2004），当CI>0.61时，日降水高度集中；有研究表明中国CI介于0.58~0.75之间，平均为0.65［10］，由此可知：云南日降水高度集中，集中程度高于中国平均水平。
在ARCGIS中绘制CI等值线图，如图2所示，总体来看，云南CI大致以大理-景东-元江-屏边为界限，东部地区日降水CI大于西部地区日降水CI，而这条界限就是对云南降水影响较大的哀牢山区。在哀牢山区分布有CI最高的元江站（CI=0.71），第二高的屏边站（CI=0.70）和大理站（CI=0.70），因此，哀牢山区是云南日降水CI的高值区域。在ARCGIS中绘制多年平均降水量等值线图，图3。在图3中可以发现哀牢山及以东地区年降水量较山脉以西地区少，冯彦等人（2010）认为哀牢山山脉阻挡了西南季风，使得东西两部的水汽输送不同，从而造成了东西两部分地区降水的差异。因此，哀牢山不但引起了东西两部分地区降水的差异，而且对日降水的集中度CI形成了影响。
图2 云南CI空间分布特征

Fig.2 The spatial distribution characteristics of CI in Yunnan

Full size|PPT slide

图3 云南多年平均降水量的空间分布特征

Fig.3 The spatial distribution characteristics of annual average precipitation in Yunnan

Full size|PPT slide

采用pearson相关系数分析CI与年降水之间的关系，相关系数为-0.50，二者具有显著的负相关关系（α=0.05），如图4（a），一定程度上，说明年降水量越少，CI越大。选择年降水量接近的景洪站和临沧站进行对比（景洪站：1 156.45 mm；临沧站： 1 145.58 mm），根据洛伦兹曲线（图5）可知，景洪站26%的降水日数、临沧站30%的降水日数都对其地区贡献了相同的76%年降水量，虽然两个站降水接近，但由于降水日数不同，两个站的CI产生差异，景洪站CI（0.69）大于临沧站CI（0.63）。进一步统计出29站贡献75%的降水量的各站降水日数，分析降水日数与CI的相关性，如图4（b）所示，CI与降水日数的相关系数为-0.93，具有显著的负相关关系（α=0.05），说明降水日数较少的地区，CI较大。Li等人（2011）在研究新疆地区CI的变化特征时也指出：降水量与降水日数较少的地区，CI明显较大。因此，年降水量和降水日数共同影响着CI。
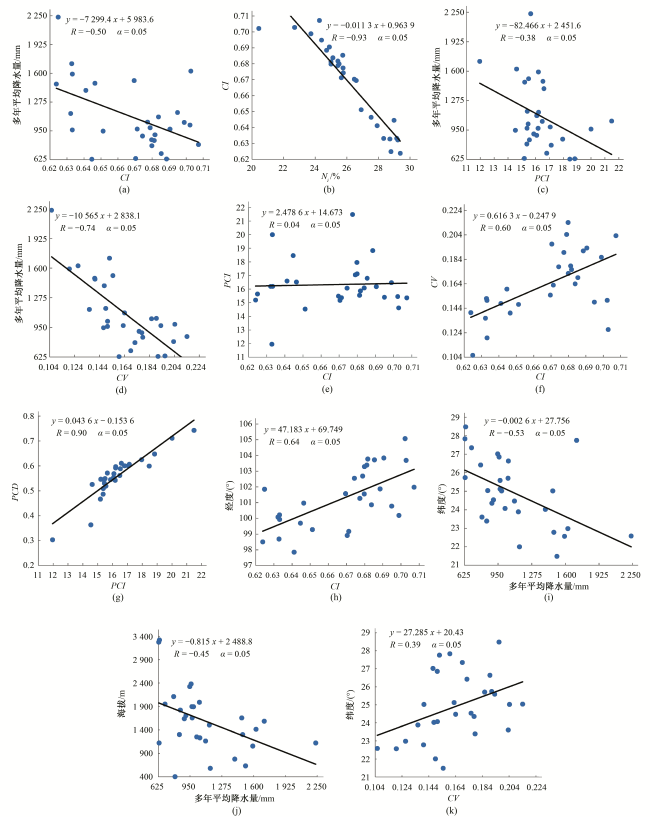
图4 各指标相关关系图（R：相关系数；α：显著性水平）

Fig.4 Correlation diagram of various indicators (R: correlation coefficient; α: significance level)

Full size|PPT slide

图5 景洪站、临沧站的洛伦兹曲线

Fig.5 Lorentz curve of Jinghong station and Lincang station

Full size|PPT slide

2.2 基于月降水的年内统计特征

由表2可知，PCI介于11.95~21.48之间，根据表3的分级标准，29站PCI均大于11，云南所有站点降水集中等级为中度集中及以上，其中3%的站点高度集中、52%的站点集中、45%的站点中度集中，故云南年内降水集中。
表3 PCI等级划分表

Tab.3 Classification of the Precipitation Concentration Index

PCI <10 11~15 16~20 >20
集中等级 均匀 中度集中 集中 高度集中
绘制PCI空间分布图（图6），云南PCI最大值为华坪站（PCI=21.48），降水高度集中，贡山站PCI最低（PCI=11.95）。总体来看，云南PCI大致可分为两部分：滇西北、滇东南的降水中度集中地区，包括德钦、景洪等13个站点；滇西南-滇中-滇东北的降水集中地区，包括中甸、昆明、昭通等15个站点。
图6 云南PCI空间分布特征

Fig.6 The spatial distribution characteristics of PCI in Yunnan

Full size|PPT slide

根据图4（c），PCI与年降水的相关系数为-0.38，具有显著的负相关关系（α=0.05）。表明降水越少，年内降水在各月份之间的分布越不均匀，各月份降水的差异性越大，降水集中在某一月份的可能性越大，年内降水集中程度越高，干旱、洪涝等自然灾害发生的概率越大。
根据表2，29站PCD介于0.30~0.74之间，华坪站PCD最大（PCD=0.74），贡山站最小（PCD=0.30），绘制PCD空间分布图（图7），总体来看，滇西北和滇东南地区PCD较小，滇北地区PCD最大，如：华坪、丽江、元谋站，其余地区PCD介于0.56~0.64之间。PCP是用来分析年内最大降水的集中时段，其中，根据表2的计算结果，59%的站点降水主要集中在7月份，41%的站点集中于8月份。
图7 云南PCD空间分布特征

Fig.7 The spatial distribution characteristics of PCD in Yunnan

Full size|PPT slide

2.3 基于CV的年际统计特征

通过计算，29站CV介于0.11~0.21之间（如表2所示），且CV最高值出现在楚雄（CV=0.21），最低值出现在江城（CV=0.11）。从CV的空间分布图来看（图8），CV呈现出由北到南逐渐减小的空间分布特征，而年降水则呈现出由西南向东北递减的分布特征，二者具有相反的空间分布特征，分析CV与多年平均降水量之间的相关关系可知［图4（d）］，CV与多年平均降水量的相关系数为-0.74，具有显著的负相关关系（α=0.05），说明年降水量越大的地区，年际变化较小。
图8 云南CV空间分布图

Fig.8 The spatial distribution characteristics of CV in Yunnan

Full size|PPT slide

2.4 CI与PCI、CV的关系

CI、PCI、CV分别从降水等级集中程度、年内集中程度和年际变化来描述降水的特征，属于不同的降水指标。
PCI、CV分别从年内、年际降水量来表征降水的变化，利用pearson相关系数分析CI与PCI、CV之间的关系，如图4（e）、4（f）：CI与PCI的相关系数为0.04，具有不显著的正相关关系（α=0.05），故CI与PCI在描述年内降水特征上无相似之处。CI与CV的相关系数为0.60，具有显著的正相关关系（α=0.05），即CI越大，CV越大，表明年际总降水量分布的不均匀性越强，年内降水在各等级上的分布越不均匀。

2.5 PCI与PCD的关系

PCI、PCD都是基于月降水来描述年内降水的集中程度，从PCI与PCD的空间分布图来看（图6、图7），二者在空间上的分布情况基本上是一致的。根据图4（g），PCI与PCD的相关系数为0.90，具有显著的正相关关系（α=0.05），表明PCI与PCD在描述年内降水的集中程度上是相似的，二者在一定程度上可以相互替换。

2.6 指标的空间分布特征

为了更好地了解云南降水的空间分布规律，利用5个指标与经纬度、海拔之间的关系来探讨云南地区降水的空间分布特征。从CI的空间分布图来看（如图2所示），哀牢山东部地区CI较西部地区大，除哀牢山对CI的影响外，经度似乎也影响着CI。利用person相关系数分析CI与经度之间的相关关系，如图4（h）所示，二者的相关系数为0.64，具有显著的正相关关系（α=0.05），故CI具有经度效应。同样地，利用person相关系数分析CI与纬度、海拔之间的关系，发现CI与纬度、海拔的相关系数分别为-0.08、-0.17，具有不显著的负相关关系（α=0.05），如表4所示，表明纬度和海拔对CI无显著的影响。
表4 各指标与经纬度、海拔相关系数表

Tab.4 Correlation coefficients of various indexes with latitude, longitude and elevation

由多年平均降水量的空间分布图（图3）可知，滇南地区的降水量明显大于滇北地区，即纬度或海拔影响着降水量。利用多年平均降水量与纬度、海拔之间的相关关系，由图4（i）、4（j）可知，年降水量随着纬度和海拔的升高呈显著下降的趋势，相关系数分别为-0.53、-0.45，表明纬度和海拔影响着总降水量。
PCI的空间分布图显示（图6），PCI随经纬度、海拔的变化无明显的分布规律，利用person相关系数分析PCI与经纬度、海拔之间的关系，如表4所示，PCI与经纬度、海拔的相关系数分别为0.09、0.22、0.15，具有不显著的正相关关系（α=0.05）。如图7所示，PCD的空间分布情况与PCI一致，PCD有可能表现出与PCI相似的空间特征。如表4所示，PCD与经度的相关系数为0.18，具有不显著的正相关关系，与纬度、海拔的相关系数分别为-0.10、-0.07，具有不显著的负相关关系（α=0.05）。另外，根据表4，PCP与经纬度、海拔的相关系数分别为-0.13、-0.27、 -0.19，具有不显著的负相关关系（α=0.05）。故PCI、PCD、PCP受经纬度、海拔的影响小，空间分布特征不明显。
CV的空间分布图表明（图8），CV由北向南逐渐减小，即降水的年际变化由北向南呈逐渐减小的趋势。利用person相关系数分析CV与纬度之间的关系，相关系数为0.39，二者具有显著的正相关关系（α=0.05），如图4（k）所示，故纬度影响CV，降水的年际变化随纬度的减小呈减小的趋势。如表4所示，CV与经度、海拔之间的person相关系数分别为0.23、0.21，表明CV与经度、海拔之间具有不显著的正相关关系，即降水的年际变化受经度、海拔影响小。

3 讨 论

CI是一个描述降水等级分布的指标，与PCI、PCD、PCP都不同，CI关注降水在某一等级上的集中程度，而PCI和PCD主要关注降水在年内时段上的集中程度。因此，CI与PCI、PCD是两类不同类型的指标，它们分别从降水等级分布和时间集中程度两个角度描述降水的特性。
PCI与PCD都是基于月降水数据来描述年内降水的集中程度，从空间分布来看，PCI在滇西北，滇东南地区最小，滇西南-滇中-滇东北地区次之，滇北地区最大，且PCI在滇北地区站点数最少（见图6）。由图7可知，PCD也表现出相同的特点（滇西北，滇东南地区最小，滇西南-滇中-滇东北地区次之，滇北地区最大且站点数最少），与PCI的分布情况是一致的，故PCI与PCD在描述年内降水集中程度上是相似的。利用pearson相关系数分析PCI与PCD之间的关系，如图4（g）所示，相关系数为0.90，二者具有显著的正相关关系（α=0.05），因此，两个指标在一定程度上是可以相互替换的。PCI是一个描述年内降水集中程度的指标，对比表3，可直接判定降水集中程度的等级，相比PCD而言，其计算简便、物理意义直观。PCP有利于描述降水集中的具体时段，从而确定降水集中的月份，这是CI和PCI两种指标不具有的特点。
CV基于年降水数据表征年际降水的分布情况，CV越大，降水的年际分布越不均匀。Royé（2017）在对美国降水集中度的研究中表明，多年平均降水量较少、纬度较大的地区，CV较大。分析云南29站CV与多年平均降水量、纬度之间的关系，相关系数分别为-0.74、0.39，故CV与多年平均降水量具有显著的负相关关系，与纬度具有显著的正相关关系（α=0.05），如图4（d）、 图4（k）所示。表明降水的年际变化随着多年平均降水量的减少、纬度的增大呈增大的趋势。

4 结 论

本文选取了云南29个气象站1960-2014年日降水数据，计算了CI、PCI、PCD、PCP、CV 5个降水统计指标，分析了各指标降水的年内、年际统计特征及各指标之间的关系，最后进一步讨论了云南地区降水的空间分布特征，结论如下。
（1）CI介于0.62~0.71之间，平均为0.67，日降水高度集中。中国的CI介于0.58~0.75之间，平均为0.65，云南年内降水的集中程度高于中国平均水平。CI与年降水量、降水日数的相关系数分别为-0.50、-0.93，具有显著的负相关关系（α=0.05），故年降水量和降水日数较少的地区，CI较大。另外，CI与经度的相关系数为0.64，具有显著的正相关关系（α=0.05），即CI具有经度效应。
（2）PCI介于11.95~21.48之间，其中，3%的站点高度集中、52%的站点集中、45%的站点中度集中。PCI与年降水量的相关系数为-0.38，具有显著的负相关关系（α=0.05），年内降水量越小，PCI越大，年内降水的集中程度越高。PCD介于0.30~0.74之间，从其空间分布图来看，各站点PCD存在明显差异，西北和东南地区PCD较小，北部地区PCD最大，其余地区PCD介于0.56~0.64之间。PCP表明了降水主要集中在7、8月份。
（3）CV与年降水量的相关系数为-0.74，具有显著的负相关关系，与纬度的相关系数为0.39，具有显著的正相关关系（α=0.05）。故降水的年际变化随着年降水量的增多、纬度的减小呈减小的趋势。年降水与海拔、纬度的相关系数分别为-0.45、 -0.53，具有显著的负相关关系（α=0.05）。空间分布上，经度影响着CI，纬度影响着总降水量，年降水量随着海拔和纬度的升高呈显著下降的趋势。
（4）CI、PCI与年降水的相关系数分别为-0.50、-0.38，呈显著的负相关关系（α=0.05）。所以不同的降水指标对于降水特征的识别，可能显现出相同的降水趋势。若年内降水越少，降水等级越集中，季节性差异越大，降水可能更容易集中在某一等级上及某一时段内，这一现象可能会加剧该地区的季节性干旱，或容易引起滑坡等灾害。另外，年际总降水分布差异越大，年内降水在某一等级上的集中程度越高。
综上，CI与PCI、PCP共同使用，既能描述降水的等级集中，又可以描述年内的集中程度和集中时段，有助于全面地描述降水的年内集中特征。

参考文献

原文顺序 | 文献年度倒序 | 文中引用次数倒序
1
BENNETTBREE， LEONARDMICHAEL， DENG Y. An empirical investigation into the effect of antecedent precipitation on flood volume［J］. Journal of Hydrology， 2018，567：435-445.
本文引用 [1]
2
QIU H， CUI Y， PEI Y， et al. Temporal patterns of nonseismically triggered landslides in Shaanxi Province， China［J］. Catena， 2019：104-356.
3
LIU Y， CHEN S， SUN H， et al. Does the long-term precipitation variations and dry-wet conditions exist in the arid areas？ A case study from China［J］. Quaternary International， 2019，519（C）：3-9.
本文引用 [2]
4
LI X， JIANG F， LI L， et al. Spatial and temporal variability of precipitation concentration index， concentration degree and concentration period in Xinjiang， China［J］. International Journal of Climatology， 2011，31（11）：1 679-1 693.
本文引用 [2]
5
REVADEKAR J V， PREETHI B. Statistical analysis of the relationship between summer monsoon precipitation extremes and foodgrain yield over India［J］. International Journal of Climatology， 2012，32（3）：419-429.
本文引用 [1]
6
PARIDA B P， MOALAFHI D B. Regional rainfall frequency analysis for Botswana using L-Moments and radial basis function network［J］. Physics & Chemistry of the Earth Parts A/b/c， 2008，33（8-13）：614-620.
本文引用 [1]
7
TFWALA C M， DVAN RENSBURG L， Schall R， et al. Drought dynamics and interannual rainfall variability on the Ghaap plateau， South Africa， 1918-2014［J］. Physics and Chemistry of The Earth， 2018，107：1-7.
本文引用 [1]
8
MARTIN‐VIDEJAVIER. Spatial distribution of a daily precipitation concentration index in peninsular Spain［J］. International Journal of Climatology， 2004，24（8）：959-971.
本文引用 [1]
9
SHI W， YU X， LIAO W， et al. Spatial and temporal variability of daily precipitation concentration in the Lancang River basin， China［J］. Journal of Hydrology， 2013，495（15）：197-207.
10
佟晓辉， 刘向培， 贾庆宇， 等. 1960-2011年中国日降水集中程度的时空变化特征［J］. 气候与环境研究，2017（3）：301-314.
本文引用 [1]
11
VYSHKVARKOVA E， VOSKRESENSKAYA E， MARTIN-VIDE J. Spatial distribution of the daily precipitation concentration index in Southern Russia［J］. Atmospheric Research， 2018， 203：36-43.
本文引用 [1]
12
SARRICOLEA P， MESEGUER-RUIZ O， SERRANO-NOTIVOLI R， et al. Trends of daily precipitation concentration in Central-Southern Chile［J］. Atmospheric Research， 2019，215（1）：85-98.
本文引用 [2]
13
ZHENG Y， HE Y， CHEN X. Spatiotemporal pattern of precipitation concentration and its possible causes in the Pearl River basin， China［J］. Journal of Cleaner Production， 2017，161（9）：1 020-1 031.
本文引用 [2]
14
PATEL N R， SHETE D T. Analyzing precipitation using concentration Indices for north gujarat agro climatic zone， india［J］. Aquatic Procedia， 2015（4）：917-924.
本文引用 [2]
15
WANG R， ZHANG J， GUO E， et al. Spatial and temporal variations of precipitation concentration and their relationships with large-scale atmospheric circulations across Northeast China［J］. Atmospheric Research， 2019，222.
本文引用 [2]
16
JOHN E OLIVER. Monthly precipitation distribution： a comparative index［J］. The Professional Geographer， 1980，32（3）：300-309.
本文引用 [1]
17
MICHIELS P， GABRIELS D， HARTMANN R. Using the seasonal and temporal Precipitation concentration index for characterizing the monthly rainfall distribution in Spain［J］. Catena， 1992，19（1）：43-58.
本文引用 [1]
18
ZHANG L J， QIAN Y F. Annual distribution features of precipitation in China and their interannual variations［J］. Acta Meteorologica Sinica，2003，17（2）：146-163.
19
CALOIERO T， COSCARELLI A R， FERRARIC B E， et al. Trend detection of annual and seasonal rainfall in Calabria （Southern Italy）［J］. International Journal of Climatology， 2011，31（1）：44-56.
20
DE LUIS M， GONZÁLEZ-HIDALGO J C， BRUNETTI M， et al. Precipitation concentration changes in Spain 1946–2005［J］. Natural Hazards and Earth System Science， 2011，11（5）：1 259–1 265.
21
COSCARELLI R， CALOIERO T. Analysis of daily and monthly rainfall concentration in Southern Italy （Calabria region）［J］. Journal of Hydrology， 2012，S 416–417：145-156.
22
DENG S， CHEN T， YANG N， et al. Spatial and temporal distribution of rainfall and drought characteristics across the Pearl River basin［J］. Ence of the Total Environment， 2018，s 619–620：28-41.
本文引用 [1]
23
ROYÉD.， MARTIN-VIDE J. Concentration of daily precipitation in the contiguous united states［J］. Atmospheric Research， 2017：237-247.
本文引用 [2]
24
LU Y J， JIANG S H， REN L L， et al. Spatial and temporal variability in precipitation concentration over mainland China， 1961–2017［J］. 2019，11（5）：881-881.
本文引用 [1]
25
冯彦， 李运刚. 哀牢山——元江河谷对区域地理分异的影响［J］. 地理学报， 2010（5）：595-604.
本文引用 [1]
PDF(4057 KB)

访问

引用

详细情况
段落导航
相关文章
鄂公网安备 42010602001274号　鄂ICP备14015464号-1
网站版权所有 © 《中国农村水利水电》编辑部
地址：武汉大学二区《中国农村水利水电》编辑部　邮编：430072
电话：(027)68776133　传真：xsdbjb@188.com　
本系统由北京玛格泰克科技发展有限公司设计开发　技术支持：support@magtech.com.cn

/

〈 〉