沙坪二级电站水力调控方法研究

李谷涵; 张召; 孔令仲; 雷晓辉; 王艺霖; 颜培儒; 许珂; Gu-han LI; Zhao ZHANG; Ling-zhong KONG; Xiao-hui LEI; Yi-lin WANG; Pei-ru YAN; Ke XU

中国农村水利水电 ›› 2022 ›› (1) : 196-199,205.

水电建设

沙坪二级电站水力调控方法研究

作者信息 +

Research on the Hydraulic Control Method of Shaping II Hydropower Station

Author information +

稿件信息 +

摘要

我国现阶段水利工程群的调控以人工经验为主，因缺乏对水流时滞性的科学分析，难以满足新时期智能调控的迫切需求。枕头坝一级~沙坪二级区间位于大渡河流域，坡度陡，水力调控研究难度大，目前主要依靠人工经验开展水力调控方案的制定。以该区域为例，利用一维非恒定流数值模拟模型，对水流时滞性进行了系统分析，计算上游流量变化、下游水位稳定时下游边界的理想流量过程，提出了一种计算快捷、操作可靠的水力调控方法，经水动力模拟验证，该调控方法产生的最大水位波动在0.2 m左右，能够保证河渠的安全。最后基于该调控方法以及敏感性分析，构建了能够快速生成沙坪二级电站不同情景下的水力调控方案的公式。

Abstract

At this stage， China’s water conservancy engineering group is still based on manual experience. Due to the lack of scientific analysis of the time lag of water flow， it is difficult to meet the urgent needs of intelligent regulation in the new era. The Zhentouba I~Shaping II section is located in the Dadu River Basin， with steep slopes and difficult hydraulic control research. At present， the hydraulic control plan is mainly based on manual experience. Taking this area as an example， this paper uses a one-dimensional unsteady flow numerical simulation model to systematically analyze the time lag of water flow， calculate the ideal flow process of the downstream boundary when the upstream flow changes and the downstream water level is stable， and a fast calculation method is proposed. The reliable hydraulic control method has been verified by hydrodynamic simulation， and the maximum water level fluctuation produced by the control method is about 0.2 m， which can ensure the safety of the river canal. Finally， based on the control method and the orthogonal test method of sensitivity analysis， a formula that can quickly generate the hydraulic control plan under different scenarios of the Shaping II Hydropower Station is constructed.

导出引用

李谷涵 , 张召 , 孔令仲 , 雷晓辉 , 王艺霖 , 颜培儒 , 许珂. 沙坪二级电站水力调控方法研究[J].中国农村水利水电, 2022(1): 196-199,205

Gu-han LI , Zhao ZHANG , Ling-zhong KONG , Xiao-hui LEI , Yi-lin WANG , Pei-ru YAN , Ke XU. Research on the Hydraulic Control Method of Shaping II Hydropower Station[J].China Rural Water and Hydropower, 2022(1): 196-199,205

0 引言

水是生命之源、生产之要、生态之基、事关人类生存、经济发展和社会进步^［1］，是人类生存和经济社会发展的重要基础资源^［2］。由于我国水资源短缺，水环境、水生态损害，水灾害频发、水环境污染等问题比较突出，必须对现有水资源进行合理利用。建设水库、拦河闸坝、节制闸、泵站、分水闸、退水闸等水力调控工程，科学合理地进行水力调控，实现水资源安全和水利工程的安全高效运行，改善推动社会、经济、生态的发展。

目前我国的明渠水利工程群仍然处于人力主观调控为主的调控阶段，难于准确控制水量且耗费人力^［3］。且明渠水流存在一定的时滞性^［4］，凭借经验的人力主观调控虽在常态情景下能够保障安全，但在处理突发情况时，缺乏科学性的经验调控极易发生重大事故。因此对明渠水力调控方法的研究就显得尤为重要。

近年来众多学者利用控制模型、算法等科学的工具，开展了对水力调控方法的研究。在积分-时滞（ID）模型^［5-7］和MPC^{控制^［8，9］算法的理论基础上，孔令仲^{［10，11］}研究分析了明渠的实时调控。崔巍、王长德等^{［12，13］}基于对调水工程控制参数取值问题的研究分析，得到最优控制方案。Zhiming Liang^［14］对黄河上游水库群进行了研究，分析了各阶段水库调度周期的影响和各流域的影响比例，制定了水库群调控的方案。上述研究针对不同的情景下水力调控方案的制定提供了方法，不过存在着一定的缺点：①模型建模过程复杂、计算量偏大，对硬件计算、存储的要求较高；②由于是连续的实时控制，需要实时滚动的收集水位数据，导致水力调控的次数过多，控制过程较频繁。}

为了获得科学且计算简便的调控方法，本文以沙坪二级电站的水力调控方法为研究对象，采用非恒定流数学控制理论，基于相邻电站间明渠水流的滞后时间研究水力调控方法，得到了该方法对应的沙坪二级电站最优调控间隔时间。同时基于对最优调控间隔时间的敏感性分析，拟合出能够适用于沙坪二级电站在各种情景下的调控时间公式。

1 研究区域概况及水动力模型构建

1.1 研究区域概况

研究区域位于大渡河流域，四川省乐山市金口河区与峨边县附近，枕头坝一级-沙坪二级区间（北纬29°13′N~29°18′N，东经103°02′E~103°12′E），流域全长28 km。

枕头坝一级水电站位于四川省乐山市金口河区，为大渡河干流水电梯级规划的第19个梯级，上一级是深溪沟水电站，下一级是枕头坝二级水电站。坝址处控制流域面积73 057 km²，多年平均流量1 360 m³/s，水库正常蓄水位624 m ^［15］。

沙坪二级水电站位于四川省乐山市金口河区，距峨边县城上游约7 km，是大渡河干流水电梯级规划的第20个梯级，上一级是沙坪一级水电站，下一级是龚嘴水电站。坝址以上流域面积73 632 km²，多年平均流量1 390 m³/s，水库正常蓄水位554 m^［16］。

本研究选取了枕头坝一级~沙坪二级河段的30个断面进行一维水动力数值模拟分析，断面的具体位置如图1所示。

图1 枕头坝一级-沙坪二级断面位置分布示意

Fig.1 Location distribution of Zhentouba I-Shaping II section

Full size|PPT slide

1.2 非恒定流水动力模型

为精确计算枕头坝-沙坪区间水流演进时间，需研发区间水动力过程精细模拟模型。

基于Visual Studio 2013平台，使用C++程序语言研究适用于库区河道的一维非恒定流水动力模型，其基本方程为Saint-Venant^［17］方程组：

B \frac{\partial Z}{\partial t} + \frac{\partial Q}{\partial x} = q_{L}

（1）

\frac{\partial Q}{\partial t} + \frac{\partial}{\partial x} (\frac{α Q^{2}}{A}) + g A \frac{\partial Z}{\partial x} + g A S_{f} = 0

（2）

式中：Z为水位，m；Q为流量，m³/s；B为过水断面表面宽度，m；A为过流面积，m²；

q_{L}

为单位长度上的旁侧入流或出流，m³/s；t为时间，s；g为重力加速度，m/s²^［18］；

S_{f}

为摩阻比降。

模型采用收敛速度快、稳定性好的Preissmann四点带权隐式差分格式^［19］离散上述方程组，利用追赶法^［20］进行求解^［21］。

1.3 上、下游边界条件及旁侧支流

在进行非恒定流河道水动力模拟时，圣维南方程的求解需要给出上、下游边界条件及沿线支流信息。上游边界条件设置为上游初始断面的流量变化过程，下游边界设置为下游末断面的水位变化过程。沿线支流信息选择位于枕头坝一级下游约2 km处的旁侧入流江沟的实测流量。

在上游流量发生变化后，为满足河道的水位稳定，需要对另一端的流量进行调控。以进口流量为例，当对流量调控过早或过晚都会导致上游调整引发的水动力动态无法平稳耦合，造成河道内水位的大幅波动，进而导致对电站运行效率和安全的威胁。为了使沙坪二级坝前控制点水位变幅最小，采取逆向分析思维，以上游流量即枕头坝出库流量作为调控序列和下游稳定水位即沙坪二级坝前稳定水位序列作为河道区间的上下游边界条件。

2 水力调控方法的确定

使用一维非恒定流水动力数值模拟模型对水力调控方法进行研究。根据2019年7月枕头坝一级平均实测出库流量 3 013 m³/s，沙坪二级平均实测坝前水位552.1 m，设定上游枕头坝一级初始流量3 000 m³/s，下游沙坪二级坝前水位552 m的初始态，计算在此初始态下，上游流量发生瞬时变化，增加300 m³/s（即上游初始流量的+10%）后，下游流量变化相应流量变化量的30%、40%、50%、60%、70%（即90、120、150、180、210 m³/s）的滞后时间，结果分别为62、67、71、76、82 min。下游流量的变化过程及发生了30 %、40 %、50 %、60 %、70 %流量变化对应的时刻如图2所示。

图2 沙坪二级出库流量变化过程

Fig.2 The process of the outflow change of Shaping II

Full size|PPT slide

随后，分别将一维水动力模型的上下游边界设置为枕头坝出库流量过程和沙坪出库流量过程：枕头坝一级初始出库流量为3 000 m³/s，在0时刻突变为3 300 m³/s；沙坪二级初始出库流量为3 000 m³/s。计算当沙坪二级电站分别在62、67、71、76、82 min 时进行调控，即增加相同流量变化量（+300 m³/s）的情景下，沙坪坝前水位的最大变幅。

在上述5种情景下进行一维非恒定流水动力数值模拟计算，计算得到沙坪二级电站在下游流量变化了上游流量变化量的30%、40%、50%、60%、70%（即62、67、71、76、82 min）时进行调控，对应的沙坪二级电站坝前水位最大水位变幅分别为26.6、23.0、20.3、22.1、29.5 cm。模拟计算得到5种情景下的沙坪二级电站坝前水位变化过程如图3所示。

图3 不同时刻进行调控的沙坪坝前水位变化过程

Fig.3 Water level change process in front of Shaping Dam under different time regulation

Full size|PPT slide

对上述模拟计算的结果进行分析，得到以下结论：

（1）过早或过晚对沙坪二级电站进行水力调控，会引发河道较大的水位波动，存在安全隐患；

（2）沙坪二级电站在下游流量变化了上游流量变化量的50 %时进行水力调控较合适，河道的水位波动较小，可以保障水库的安全运行。

3 参数敏感性分析的正交试验法

为使水力调控方法更具快捷性，基于上一节的研究分析，设定下游流量变化了上游流量变化量50 %的时间为最优调控间隔时间。本节将基于正交试验法分析最优调控间隔时间对上游初始流量、下游水位和流量变化量变化的敏感性，为得到能够适用于不同情景下的水力调控方法提供支撑。

3.1 正交试验设计

正交试验方法以概率论、数理统计和实践经验为基础，采取部分试验来代替全部试验的方法，通过对结果进行分析，了解全面试验的情况^{［22， 23］}。由于正交表具有均衡分散性和整齐可比性的构造原则，因此，此方法设计的试验次数少，且能反映客观事物的基本规律。

选择三因素三水平试验，考虑影响最优调控间隔时间的三个因素，即上游初始流量、下游水位、流量变化量，并对每个因素设计三个水平，水平表如表 1所示。水平的选取充分考虑到了丰、平、枯水期的枕头坝一级出库流量、沙坪二级坝上水位的范围。

表1 最优调控间隔时间因素水平表

Tab.1 The level table of the optimal regulation interval time factor

水平	因素
水平	上游初始流量/（m³·s^-1）	下游水位/m	流量变化量/%
水平1	1 000	552	-10
水平2	2 000	553	-20
水平3	3 000	554	-30

为减少试验次数，提高试验效率，同时又能充分反映各因素对分析结果的影响，选用L₉（3⁴）正交表进行试验设计^［24］，并计算相应的最优调控间隔时间，结果如表2所示。

表2 最优调控间隔时间正交试验表

Tab.2 Orthogonal experimental table of optimal control interval time

试验号	因素			试验结果
	A	B	C	试验结果
	上游初始流量/（m³·s^-1）	下游水位/m	流量变化量/%	最优调控间隔时间/min
1	1 000	552	-10	95
2	1 000	553	-20	95
3	1 000	554	-30	96
4	2 000	552	-30	87
5	2 000	553	-10	85
6	2 000	554	-20	84
7	3 000	552	-20	80
8	3 000	553	-30	79
9	3 000	554	-10	75

3.2 试验结果极差分析

极差分析法常被用来处理和分析正交试验的结果，通过极差分析，可对多因素问题进行参数敏感性分析，确定主次影响因素。极差较大的因素是主要影响因素，较小的是次要影响因素^［25］。对试验结果表2进行极差分析，结果如表3所示，表中K ₁、K ₂、K ₃分别为同一因素下相同水平试验结果的总和、k ₁、k ₂、k ₃分别为K ₁、K ₂、K ₃的平均值，R_j 为不同因素的极差。上游初始流量的极差R_j 为5.78，远大于下游水位、流量变化量的极差0.78，由此可知最优调控间隔时间对上游初始流量的变化较敏感，而对下游水位、流量变化量的变化不敏感。

表3 各影响因素不同水平作用下极差分析结果

Tab.3 Results of range analysis under different levels of influence factors

项目	上游初始流量/（m³/s）	下游水位/m	流量变化量/%
K ₁	95.33	87.33	85.00
K ₂	85.33	86.33	86.33
K ₃	78.00	85.00	87.33
k ₁	31.78	29.11	28.33
k ₂	28.44	28.78	28.78
k ₃	26.00	28.33	29.11
R_j	5.78	0.78	0.78

4 最优调控间隔时间公式拟合

本节基于参数敏感性分析的结论，构建最优调控间隔时间与各类水力要素间的最优函数关系，从而实现不同情景下水力调控方案的快速生成，让该方法更加快捷。由于最优调控间隔时间对上游初始流量的变化较敏感，对下游水位、流量变化量的变化不敏感，考虑上游初始流量对最优调控间隔时间的影响，而忽略下游水位、流量变化量微弱的影响，拟合最优调控间隔时间与上游初始流量之间的关系。

控制下游水位为正常蓄水位554 m，流量变化量为上游初始流量的-10%不变。考虑枕头坝一级出库在丰水期的大流量、枯水期的小流量以及平水期流量的范围，计算上游初始流量分别为500、1 000、2 000、3 000、4 000 m³/s的工况下对应的最优调控间隔时间，并基于最小二乘法回归分析^{［26， 27］}的方法，试选用二次多项式、线性和乘幂关系拟合最优调控间隔时间与上游初始流量之间的函数关系，得到如图4所示结果，拟合优度R ²分别为0.998 7、0.916 9、0.981 9。因此选用优度最高的二次多项式关系拟合得到如式（3）所示最优调控间隔时间与上游初始流量的函数关系，并通过该函数关系实现水力调控方案的快速生成。

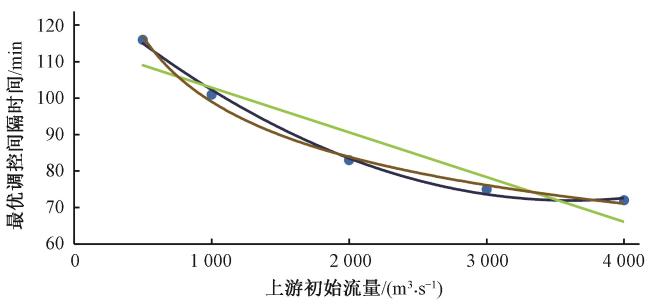
图4 最优调控间隔时间与上游初始流量拟合结果

Fig.4 Fitting results of optimal regulation interval time and upstream initial flow

Full size|PPT slide

T = 3.84 \times 10^{- 6} Q^{2} - 2.96 \times 10^{- 2} Q + 128.35

（3）

式中：T为最优调控间隔时间，min；Q为上游初始流量，m³/s；Z为下游水位，m。

5 结论

本文使用一维非恒定流水动力数值模拟模型，基于明渠水流的时滞性，提出了上游发生瞬时流量变化后，沙坪二级电站在下游发生了相应流量变化量50%的流量变化时，进行等同于上游流量变化量的水力调控的方法。并通过参数敏感性分析的正交试验法，分析了上游初始流量、下游水位、流量变化量的变化对最优调控间隔时间的影响程度，得出了最优调控间隔时间对上游初始流量的变化较敏感，而对下游水位、流量变化量的变化不敏感的结论。因此考虑上游初始流量对最优调控间隔时间的影响，而忽略下游水位、流量变化量微弱的影响，使用最小二乘法回归分析的方法，最终拟合得到可以快速生成沙坪二级电站不同情景下的水力调控方案的公式。该调控方法具有计算快捷的优势，同时通过水动力模拟验证，该调控方法产生的最大水位波动在0.2 m左右，能够保证河渠的安全，但要推广至其他工程，还需进一步探讨与研究。 □

参考文献

原文顺序 | 文献年度倒序 | 文中引用次数倒序

1	王浩，王建华. 中国水资源与可持续发展［J］. 中国科学院院刊，2012，27（3）：352-358，331. 本文引用 [1]

2	王浩，贾仰文. 变化中的流域“自然-社会”二元水循环理论与研究方法［J］. 水利学报，2016，47（10）：1 219-1 226. 本文引用 [1]

3	王浩，雷晓辉，尚毅梓. 南水北调中线工程智能调控与应急调度关键技术［J］. 南水北调与水利科技，2017，15（2）：1-8. 本文引用 [1]

4	孔令仲. 大型明渠输水工程常态控制与应急调控算法研究［D］. 杭州：浙江大学，2019. 本文引用 [1]

5	LITRICO X， FROMION V. Tuning of robust distant downstream PI controllers for an irrigation canal pool. I： Theory ［J］. Journal of Irrigation and Drainage Engineering， 2006，132（4）：359-368. 本文引用 [1]

6	LITRICO X， FROMION V， BAUME J P， et al. Experimental validation of a methodology to control irrigation canals based on Saint- Venant equations ［J］. Control Engineering Practice，2005，13（11）： 1 425-1 437.

7	ERMOLIN Y A. Study of Open-Channel Dynamics as Controlled Process［J］. Journal of Hydraulic Engineering，1992，118（1）：59-72. 本文引用 [1]

8	邱训平，杨丽莉. 地表水水量和水质的联合实时控制［J］. 水利水电快报，2012，33（11）：1-5. 本文引用 [1]

9	XU M. Real-time Control of Combined Water Quantity & Quality in Open Channels［D］. Delft： Delft University of Technology，2013. 本文引用 [1]

10	孔令仲，王浩，雷晓辉，等. 基于线性预测控制算法的明渠实时控制研究［J］. 水利学报，2020，51（3）：326-334. 本文引用 [1]

11	杨迁，孔令仲，宋培兵，等. 耦合前馈控制策略的明渠线性最优控制方法［J］. 水力发电学报，2020，39（5）：64-71. 本文引用 [1]

12	崔巍，王长德. 调水工程运行最优控制研究［J］. 南水北调与水利科技，2007（2）：6-8，24. 本文引用 [1]

13	王长德，张礼卫. 下游常水位水力自动控制渠道运行动态过程及数学模型的研究［J］. 水利学报，1997（11）：12-20，71. 本文引用 [1]

14	ZHIMING L， GAO F， ZHANG H， et al. Research on the impact of upstream reservoirs regulation on the operation of Xiluodu Reservoir［C］. MATEC Web of Conferences，2018，246. 本文引用 [1]

15	熊玺，周林勇. 混凝土缺陷水下修复技术在枕头坝一级水电站的应用［J］. 水电与新能源，2018，32（04）：56-58. 本文引用 [1]

16	涂承义，黄维，吴宏荣，等. 沙坪二级水电站预应力闸墩设计及三维有限元分析［J］. 人民长江，2019，50（）：108-113，134. 本文引用 [1] 摘要增刊2

17	麦赫默德，叶夫耶维奇. 明渠不恒定流［M］ . 北京：水利电力出版社， 1987. 本文引用 [1]

18	NEWTON. Mathematical principles of natural philosophy and his system of the world［M］. University of California Press， 1962. 本文引用 [1]

19	MU J， ZHANG X. Real-time flood forecasting with 1-D unsteady flow model［J］. Journal of hydrodynamics， 2007，19（2）：150-154. 本文引用 [1]

20	WANG C， YANG J， NILSSON H. Simulation of Water Level Fluctuations in a Hydraulic System Using a Coupled Liquid-Gas Model［J］. WATER，2015，7（8）：4 446-4 476. 本文引用 [1]

21	王船海，李光炽，向小华，等. 实用河网水流计算［M］. 河海大学出版社，2015. 本文引用 [1]

22	刘瑞江，张业旺，闻崇炜，等. 正交试验设计和分析方法研究［J］. 实验技术与管理，2010，27（9）：52-55. 本文引用 [1]

23	李凡琦，牧振伟，孙德旭，等. 基于正交设计的糙条消能工评价方法［J］. 排灌机械工程学报，2020，38（5）：481-487. 本文引用 [1]

24	方开泰，马长兴. 正交与均匀试验设计［M］. 北京：科学出版社，2001. 本文引用 [1]

25	张旭辉，龚晓南，徐日庆. 边坡稳定影响因素敏感性的正交法计算分析［J］. 中国公路学报，2003（1）：37-40. 本文引用 [1]

26	何晓群. 现代统计分析方法与应用［M］. 北京：中国人民大学出版社， 1998. 本文引用 [1]

27	梅长林. 数据分析方法［M］. 北京：高等教育出版社，2006. 本文引用 [1]

PDF(1808 KB)

1209

访问

引用

详细情况

段落导航

收稿日期	出版日期
2021-04-06	2022-01-15
发布日期
2022-02-13

选择文件类型/文献管理软件名称

选择包含的内容